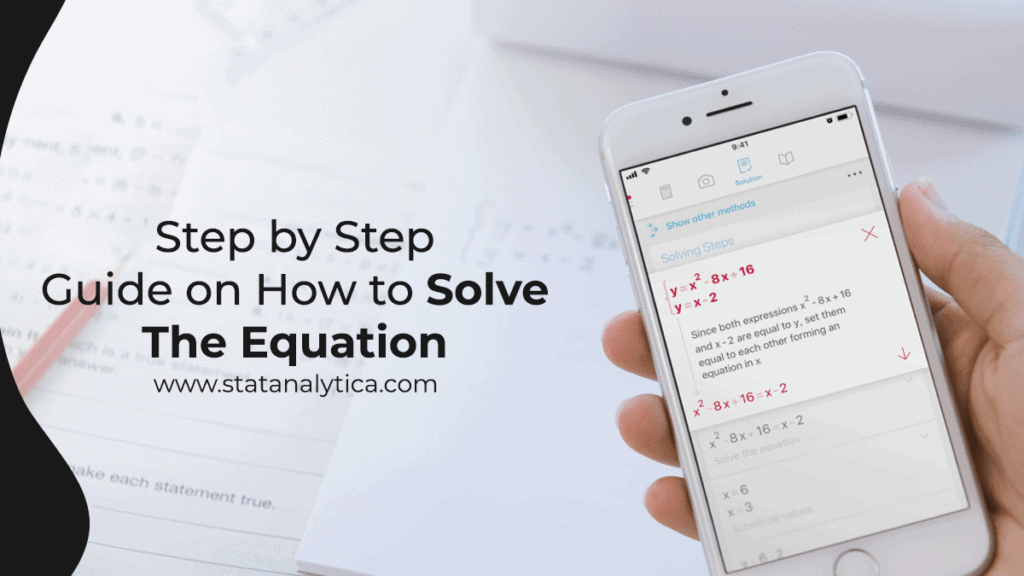
Numerous students believe that algebra and equations are beyond their understanding level. Therefore, this thought of solving the variable equations can cause a fear in them. However, it does not require to get afraid of these equations. The great news can be that these equations are comparatively simple notions. With minimal practice and the use of various simple formulas, the students can master to manage and solve the equations. This post will help the learners to understand the steps for how to solve the equation efficiently. Let’s check some more details about algebra equations.
What are the equations?
Table of Contents
An equation can be one or two variables on both sides of a number, which symbolizes their connection. That connection can be less than (<), equals (=), or greater than (>), or any order. For instance, more than or equal to (>=) or approximately equal to (≈) or even not equal to (≠). These are called equality figures. The simple equations can be defined as 4 + 4 = 8 and 6 + 2 < 5 + 5.
But, while several individuals talk regarding the one or more variables equations, they express algebraic variable equations. These equations might compose numbers as well as letters. Variables can replace by some of the numeric values where a mathematical definition could be too complex. This is the place where the students like to conclude instead of using particular products. One can also utilize it if they understand the conditions in the section, but other values are unexplained, and the students require to operate them. This is how to solve the equation effectively.
Elementary Algebraic terms
Elementary Algebraic terms include easy norms and regulations on numeric quantities such as:
- Subtraction
- Addition
- Multiplication
- Equation solving techniques
- Division
- Variables
- Polynomials
- Functions
- Algebraic Expressions
It does not have any limit on the complications of these basics besides “Algebra” is the main concern. More different theories and ideas can add to improve the level of knowledge. All those ideas can be beneficial and easy-to-learn if taught effectively.
Algebra equations are determined by operating what numeric quantity can be represented in the letters. Students can utilize the two simplistic equations over the algebraic values by replacing x for a single number:
4 + 4 = a
It is known to all that 4 + 4 = 8, which implies that a should be equal to 8. The answer to the given equation is a = 8. This can be an effective way for how to solve the equation.
6 + 3 < 5 + a
It is known to all that 6 + 3 = 9. The comparison shows the conclusion that 9 is less than (<) 5 + a. One requires rearranging the given equation so that a is on an individual side, and all the quantities are on the opposite side. Otherwise, the students face difficulty to get the value of a. The norm of replacing equations is what the students can do to one side; it must be done on the opposite side. Deduct 5 from each side (9 − 5 = 4) , then the given equation will symbolize 4 < a. Here we can recognize that a should be greater than (x < 4).
Now, one may not say more specifically what a is including the data that one is provided. However, one can observe in the first equation that there is an example where we replaced 8 for a, which is certainly greater than 4.
It is not magic that one is practicing a curly ‘a’ (a). Students can utilize any alphabet they like, although a and x are usually practiced to describe the unfamiliar components of the given equations.
Techniques for how to solve the equation
Learn the golden rule
The prime step for how to solve the equation is to get the variables on the single side or L.H.S./R.H.S., and other numeric numbers must be on the opposite side. Practice and learn this algebraic golden rule: What students can do to simplify the complicated equation is to take variables aside. This is how equations can become easy to solve.
Start with simple questions then move to complicated questions
“Practice makes a man perfect” therefore, one should start with the less complex equation. The primary algebra equation must contain some easy subtraction or addition with an individual or multiple variables, like x+11 = 13. Methods to solve the value of x with itself? Deduct 11 from each side: x + 11 -11 = 13 -11. Now solve the value by taking the simple mathematics operation: x + 11-11 = 13 – 11, or x = 2. Students can verify their results by using the substitution method. Put the value 2 in the equation in the place of x. Does 2 + 11 = 13? Yes, it is; therefore, we can say that the correct solution is x=2. This is how one can find an answer to how to solve the equation.
Conclusion
In conclusion, the mathematician defines the methods about how to solve the equation. Moreover, we have mentioned details about algebraic equations that help students solve mathematics in their daily lives. Besides this, we have provided solutions with detailed examples. So that students can easily understand the techniques and implement them to solve variables. Analyzing these examples can help the students to know the sequence of solving a variable equation. Follow the steps as mentioned above to get the desired result of the variable and verify it accordingly. Learn and practice the initial rule to solve each problem of algebra in single or multiple variables. If you still find it difficult to do math homework then you can ask us to help me with my math homework and get help from the math homework helpers.