Are you looking for the best method to lose weight? If yes!! Let’s know how to solve fractions. Sounds weird? Yes, you read it right! You need to know Body Mass Index (BMI) using fractions to lose weight effectively.
Ladies, does the jeweler give you 18 or 24-karat jewelry? 24 karats are considered pure gold, whereas 18 karats mean 18/24, equal to 75% gold. This is how you can use fractions to know jewelry purity.
This blog will help you to understand how to solve fractions using various methods. Moreover, I have listed some useful tips to use fractions. So, without creating more confusion, let’s understand the concept of fractions in detail.
Necessary things to know about Fraction!!
Table of Contents
First, understand what fraction is.
Fraction is basically a numerical quantity or value that is not a whole number, for example, 4/5, 6/7, etc.
| Fun Fact: There is a fact that helps you impress your teachers and friends. That is the bar or the division sign in the fraction known as “VINCULUM.” |
The next thing to learn is the terms used for infractions. So each and every fraction has two parts.
| -> The first part is called a numerator written at the top of the division symbol, and it makes the number of parts of the fraction equal. In short, it signifies the number of parts we have in fractions. | -> Whereas the second part is called a denominator written below the division symbol, it basically equals the total number of parts in a whole, and this is why it signifies the total parts of the number in the whole. |
For example, In the 3/5 fraction, 3 is the numerator, and 5 is the denominator.
Here 3 states that it is the 3rd part of the whole number, and 5 is signifying that the whole number has 5 parts.
The second thing to learn about types of fractions.
There are three types of fractions that we deal with. Let’s check each of them one by one.
- Proper fraction: In this fraction, the numerator has less value as compared to the denominator.
| Note: The value of the proper fraction is always less than 1. | Example of the proper fraction is 3/5, 1/2, and 5/7. |
- Improper fraction: In this fraction, the numerator is always greater than the denominator.
| Note: The value of the improper fraction is always more than 1. | The example of improper fraction is 6/3, 50/21, and 16/25. |
- Mixed fraction: In this fraction, the number is represented as the whole number, which is followed by the fractional numbers.
| Note: The mixed fraction is also called a mixed number. | Example of the mixed fraction is 6⅘, 2⅔, and 25⅝. |
How to represent fractions in different forms?
Representing the mixed fraction to the whole number.
Let’s understand the steps to solve mixed fractions to the whole number with an example.
Change 7⅘ into the whole number.
| -> First, multiple a whole number with the fraction’s denominator. That is 7 * 5 =35. |
| -> Now, add the resulting value to the numerator of the fraction. That is 35 + 4 = 39. |
| -> Take the numerator and put it in the original denominator. That is 39/5. |
| -> The final whole fraction will be 39/5. |
Representing the fraction to the decimal number.
The easiest method to change the fraction into decimal is just by dividing the number. Here, you require to divide the numerator with the denominator.
Change the fraction 7/10 into decimal.
| You need to just divide the numerator 7 by the denominator which is 10. You get the answer that is: |
| 7 /10 => 0.7 |
Representing the fraction to the percentage.
Three methods can be used for converting the fraction to a percentage. Below, I have given three different methods taking the example 7/20.
| Divide the numerator by the denominator and multiply the result by 100. |
| That is: 7/20 = 0.350.35 * 100 = 35% |
| Multiply the numerator by 100, and divide the number by the denominator. |
| 7 *100 = 700700/20 = 35% |
| Divide the numerator by the denominator and change the decimal point to the right of two places. |
| 7/20 = 0.35 Now, move the decimal points to convert them into 35%. |
Tutorial step-wise – How to solve Fractions
Let’s first learn how to add two or more fractions with the help of an example.
Suppose you have to add 3/4 with 1/4.
Here you can see the denominators are the same, so it is the simplest addition in fractions.
So the first step in how to solve fractions by addition is to find the common denominators of the numbers. In this problem, both have the same denominator, so the common between them is 4 only.
So you can write the equation as follows –
3/4 + 1/4
= (3+1)/ 4
= 4/4 = 1
If you have different denominators, then also you easily solve the fractions. Let’s learn through different example –
Suppose you have to add 3/4 with 2/5, then you will have the following equation –
3/4 + 2/5
Then the next step to solving this fraction in how to solve fractions is to find out any common denominator.
Since it has no common denominator, we will multiply both the denominators and add the above numbers. Look at the below to understand better.
= (3+2) / 4×5
= 5/20
=1/4
Now let’s take one more example where we can find the common denominators.
Suppose the fractions we need to add are ¾ and ⅝
= 3/4 + 5/8
Now we will find the least common factor that is LCM between the two denominators.
Then we will get 8 as LCM, and thus the equation will be as follows –
= (3×2 + 5)/ 8
Since the denominator is 8 and the denominator of the first fraction is 4, so we will multiply the first fraction with 2 to make the denominator of the fraction is 8; thus, the equation will be
= (6+5)/8
= 11/8
So the answer is 11/8.
Now let’s learn the subtraction of fractions in how to solve fractions.
Suppose the equation is 3/2 – 1/2
So we will follow the same process, and we will first bring out the common factor since the denominator is the same in this equation, so there is no such issue.
Now you can directly put the 2 as a denominator and then subtract the 1 from 3. Follow the steps –
=(3-1)/2
=2/2
= 1
We have got the answer that is 1.
Another example of how to solve fractions
5/7 – 2/4
We can see no common factor between the denominators, so we make the denominators the same by multiplying the first fraction with 4 and the second fraction with 7; then, we will get the following equation.
= 5×4/7×4 – 2×7/4×7
We have to make common denominators in order to solve the equation, as then only we can perform the Luther operations in the equation. Thus we will get the following answer –
=(20-14)/7×4
= 6/28
Now we can see 2 is common to both numerator and denominator, so we can divide the whole fraction with 2 in the following way –
=6\2 /28\2
= 3/14
Thus the answer is 3/14.
Now let’s learn how to multiply two fractions. This is also very important to learn how to solve fractions.
Let’s take the following example –
¾ x ⅕
Multiplication is very easy in fractions as you just have to multiply numerators with each other and likewise denominators with each other.
Then you will get the following result –
= 3×1 / 4×5
=3/20
Thus the answer is 3/20.
Now let’s learn the method of division in fractions in how to solve fractions.
You can take the reciprocal of the fraction to divide the fraction. To reciprocate, you need to switch the denominator to the numerator and the numerator to the denominator.
Let’s take an example of it:
Solve the 1/2 ÷ 1/5.
First, take the reciprocal of 1/5 as 5/1.
Take the reciprocal fraction and multiply it with another number (s).
1/2 * 5/1
To solve it, multiply the denominators and numerators:
2 * 1 = 2 (denominator)
1 * 5 = 5 (numerator)
That is : 1/2 * 5/1 = 5/2 = 2.5
Things to understand to avoid common mistakes in fractions!
It might not be easy to add and subtract the fractions with different denominators. That is why various students fail to solve the fractions with different denominators and make some mistakes.
First, let’s an example of solving fractions with different denominators.
| => Solve 3/4 + 1/6. -> The first thing that you have to do is multiply the numerator and denominator with the opposite denominator value. -> That is multiple 4 with 6 and 6 with 4. It will give you 24 in the denominator. -> Similarly, multiply numerator as 3*6 = 18 and 1*4 = 4. -> Now add 18 + 4 = 22. -> It means that 3/4 + 1/6 = 22/24 => 11/12 |
Now, understand what kind of mistakes students make.
- Misunderstand the requirements of the questions, such as dividing instead of multiplying and so on.
- When students need to add or subtract a fraction, they forget to change the fraction’s denominator. [Like in the above examples 4 and 6 change to 24].
- Moreover, it is noticeable that the numerator also needs to change as that of the denominator. [Like 3 * 6 =18 and 1 * 4 = 4].
- Finally, some students are unable to simplify the equation. [Like 22/24 also written as 11/12 after dividing the number by 2].
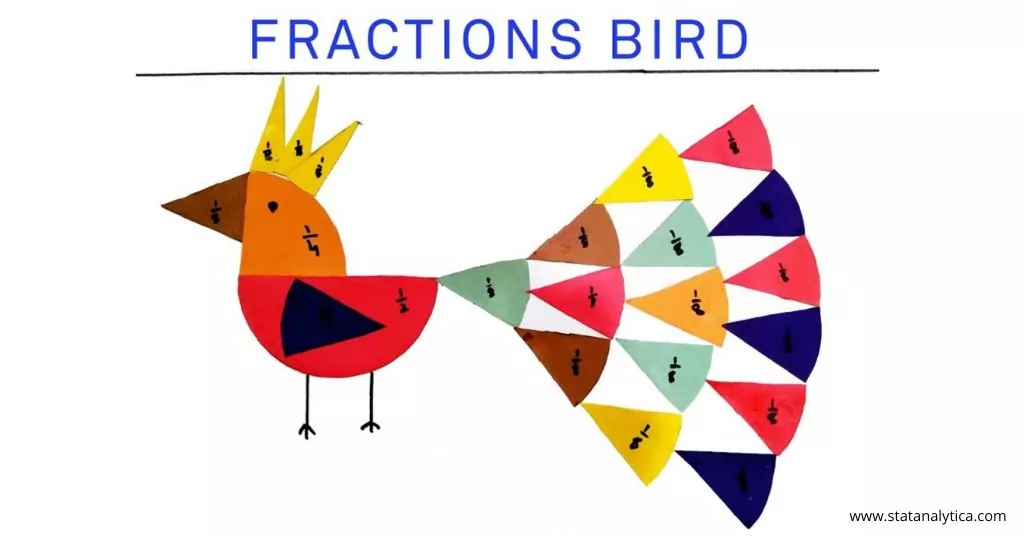
| A creative activity to teach your children about fractions!! You can teach your young ones about fractions by asking them to draw a bird using paper. Give them instructions to cut the paper in the given proportion. -> Provide them with a circle and teach them about fractions. -> Cut the circle into 8 parts and teach the fractions like 1/8, 2/8, 3/8, 4/8, 5/8, 6/8, 7/8, and 8/8. -> Also, allow them to simplify the fractions as 2/8 => 1/4, 4/8 => 1/2, 6/8 => 3/4, and 8/8 => 1. -> Finally, they have to draw the bird as: |
Conclusion
Many students are struggling with fractions, and fractions look tough at first instance but are easy when you practice them on a daily basis.
If you are also searching for how to solve fractions, then we hope that this article would have helped you in understanding the process of solving fractions. If still, you are facing difficulty in solving fractions, then you can contact us anytime. We are always here to help you. Our professionals will give you 24/7 guidance. Get the best math homework help from the experts.
Follow the link for additional details
Frequently Asked Questions
Q1. What is the formula for fractions?
Fraction = selected number of parts / total number of parts
Each fraction has a numerator, which is equal to the selected number of parts, and a denominator is equal to the total number of parts as a whole.
Q2. What is A and B in fraction?
In the fraction, A and B are considered as A/B. Where the number A is the numerator, whereas the B is the denominator.
Q3. What is 1/3 equivalent to as a fraction?
The 1/3 fraction is equivalent to: 2/6, 3/9, 4/12, 5/15, 6/18, 7/21, 8/24, 9/27, 10/30, and so on.